In this video, we take a look at experimental error. Now experimental error is connected to 2 other concepts we've discussed before in the past, the ideas of accuracy and precision. Now, we're going to say when any calculation is done, there is a level of error involved. We're going to say error itself can be grouped into 2 major categories. We have our random errors and we have our systematic errors. We're going to say when it comes to random error, random error is basically the lack of precision. And remember precision is how close your results are to one another. So if you take 4 measurements and they're all close to one another, then you are precise. Systematic error is a lack of accuracy. Now you can have 4 measurements that are close to one another and therefore are precise, but they may not be accurate if they're far away from the actual value. So accuracy is how close you can get to the actual agreed-upon value for an object when it comes to measuring it. Now we're going to say here that random errors are unpredictable and can lead to results that are either too high or too low. Basically, as the student within the lab, you have basically no control over random errors. It could be the result of random occurrences that happen just in unperceived ways. So it's really hard to minimize these types of errors. Now when we say it could be either too high or too low that means that if I, let's say, measure out an object and it is 1 gram too heavy, then all of a sudden it's 2 grams too light. And it fluctuates back and forth between being either too heavy or too light in terms of its mass. This would be an example of random error. Now here's the thing. Because our results can either be too high or too low, the best way to approach random error is to take several measurements over time. If you take several measurements and then average out all those measurements that will help to reduce your random error. Systematic error is different. Systematic errors are more predictable and can lead to results that are always too high or always too low, but not both. So let's say I weigh an object and it's consistently 1 gram too heavy. That is a systematic error. The error is always too high. Or I use another scale and I measure out something and it's consistently 2 grams too light. And it's always that. No matter what object I measure, it's always 2 grams less. That's a systematic error. That's something that I can basically, adjust and expect to occur because I know it's going to be 2 grams too light each time. But here's the thing. Whereas random errors are easier to spot because you'll get measurements that are too high and too low randomly, systematic errors are harder to spot. You may not know that you have a systematic error because let's say you take that object and you measure it several times and it keeps giving you that same exact value. You think that you are accurate. But in reality, the scale is giving you numbers that are 2 grams less than what they should be. You wouldn't know that. Now in most cases, a percent error of less than 10% will be acceptable. So if you're doing your experiment, you can test for your percent error and see is it within these guidelines. If so, it will be an acceptable answer. Percent error itself is experimental value minus theoretical value in absolute brackets which means that even if you get a number that's negative inside of here, because it has absolute brackets it'll be a positive value. And then it's divided by your theoretical value and you multiply it times 100. Now remember this times 100 is what gives us our percentage value, similar to mass percent and other concepts that we've talked about in other chapters. We're going to say the percent error formula is a useful tool for determining the precision of your calculations. Huge differences in percent error, huge values in percent error means that your numbers are not very precise. Now we're going to say here that the experimental value is your calculated value. You do the experiment, you crunch some numbers, you do the math, you get this total. That's your experimental value. You say, "I'm weighing out an object." And in the literature, in your manual, it says that it should weigh 25 grams. In the literature, it tells you that's the weight it should be so that is your theoretical value. You weigh it out a bunch of times and you get 24.8 grams. That is your experimental value. You'd plug those in and see what your percent error is. Since the numbers are pretty close to one another, it's safe to assume that your percent error wouldn't be that high. So it looks like it would be somewhat of an acceptable value. Now now that we've seen the different classifications of error, let's see if you guys can tackle these example questions. Attempt to do the first one on your own, but if you get stuck, don't worry. Just come back and take a look at my explanation on the best answer here. Here, I've done it as 1, 2, 3, and 4, meaning that more than one answer could be the correct choice. So pay attention to what we talked about up above to answer this question on random errors.
- 1. Matter and Measurements4h 29m
- What is Chemistry?5m
- The Scientific Method9m
- Classification of Matter16m
- States of Matter8m
- Physical & Chemical Changes19m
- Chemical Properties8m
- Physical Properties5m
- Intensive vs. Extensive Properties13m
- Temperature (Simplified)9m
- Scientific Notation13m
- SI Units (Simplified)5m
- Metric Prefixes24m
- Significant Figures (Simplified)11m
- Significant Figures: Precision in Measurements7m
- Significant Figures: In Calculations19m
- Conversion Factors (Simplified)15m
- Dimensional Analysis22m
- Density12m
- Specific Gravity9m
- Density of Geometric Objects19m
- Density of Non-Geometric Objects9m
- 2. Atoms and the Periodic Table5h 23m
- The Atom (Simplified)9m
- Subatomic Particles (Simplified)12m
- Isotopes17m
- Ions (Simplified)22m
- Atomic Mass (Simplified)17m
- Atomic Mass (Conceptual)12m
- Periodic Table: Element Symbols6m
- Periodic Table: Classifications11m
- Periodic Table: Group Names8m
- Periodic Table: Representative Elements & Transition Metals7m
- Periodic Table: Elemental Forms (Simplified)6m
- Periodic Table: Phases (Simplified)8m
- Law of Definite Proportions9m
- Atomic Theory9m
- Rutherford Gold Foil Experiment9m
- Wavelength and Frequency (Simplified)5m
- Electromagnetic Spectrum (Simplified)11m
- Bohr Model (Simplified)9m
- Emission Spectrum (Simplified)3m
- Electronic Structure4m
- Electronic Structure: Shells5m
- Electronic Structure: Subshells4m
- Electronic Structure: Orbitals11m
- Electronic Structure: Electron Spin3m
- Electronic Structure: Number of Electrons4m
- The Electron Configuration (Simplified)22m
- Electron Arrangements5m
- The Electron Configuration: Condensed4m
- The Electron Configuration: Exceptions (Simplified)12m
- Ions and the Octet Rule9m
- Ions and the Octet Rule (Simplified)8m
- Valence Electrons of Elements (Simplified)5m
- Lewis Dot Symbols (Simplified)7m
- Periodic Trend: Metallic Character4m
- Periodic Trend: Atomic Radius (Simplified)7m
- 3. Ionic Compounds2h 18m
- Periodic Table: Main Group Element Charges12m
- Periodic Table: Transition Metal Charges6m
- Periodic Trend: Ionic Radius (Simplified)5m
- Periodic Trend: Ranking Ionic Radii8m
- Periodic Trend: Ionization Energy (Simplified)9m
- Periodic Trend: Electron Affinity (Simplified)8m
- Ionic Bonding6m
- Naming Monoatomic Cations6m
- Naming Monoatomic Anions5m
- Polyatomic Ions25m
- Naming Ionic Compounds11m
- Writing Formula Units of Ionic Compounds7m
- Naming Ionic Hydrates6m
- Naming Acids18m
- 4. Molecular Compounds2h 18m
- Covalent Bonds6m
- Naming Binary Molecular Compounds6m
- Molecular Models4m
- Bonding Preferences6m
- Lewis Dot Structures: Neutral Compounds (Simplified)8m
- Multiple Bonds4m
- Multiple Bonds (Simplified)6m
- Lewis Dot Structures: Multiple Bonds10m
- Lewis Dot Structures: Ions (Simplified)8m
- Lewis Dot Structures: Exceptions (Simplified)12m
- Resonance Structures (Simplified)5m
- Valence Shell Electron Pair Repulsion Theory (Simplified)4m
- Electron Geometry (Simplified)8m
- Molecular Geometry (Simplified)11m
- Bond Angles (Simplified)11m
- Dipole Moment (Simplified)15m
- Molecular Polarity (Simplified)7m
- 5. Classification & Balancing of Chemical Reactions3h 17m
- Chemical Reaction: Chemical Change5m
- Law of Conservation of Mass5m
- Balancing Chemical Equations (Simplified)13m
- Solubility Rules16m
- Molecular Equations18m
- Types of Chemical Reactions12m
- Complete Ionic Equations18m
- Calculate Oxidation Numbers15m
- Redox Reactions17m
- Spontaneous Redox Reactions8m
- Balancing Redox Reactions: Acidic Solutions17m
- Balancing Redox Reactions: Basic Solutions17m
- Balancing Redox Reactions (Simplified)13m
- Galvanic Cell (Simplified)16m
- 6. Chemical Reactions & Quantities2h 35m
- 7. Energy, Rate and Equilibrium3h 46m
- Nature of Energy6m
- First Law of Thermodynamics7m
- Endothermic & Exothermic Reactions7m
- Bond Energy14m
- Thermochemical Equations12m
- Heat Capacity19m
- Thermal Equilibrium (Simplified)8m
- Hess's Law23m
- Rate of Reaction11m
- Energy Diagrams12m
- Chemical Equilibrium7m
- The Equilibrium Constant14m
- Le Chatelier's Principle23m
- Solubility Product Constant (Ksp)17m
- Spontaneous Reaction10m
- Entropy (Simplified)9m
- Gibbs Free Energy (Simplified)18m
- 8. Gases, Liquids and Solids3h 25m
- Pressure Units6m
- Kinetic Molecular Theory14m
- The Ideal Gas Law18m
- The Ideal Gas Law Derivations13m
- The Ideal Gas Law Applications6m
- Chemistry Gas Laws16m
- Chemistry Gas Laws: Combined Gas Law12m
- Standard Temperature and Pressure14m
- Dalton's Law: Partial Pressure (Simplified)13m
- Gas Stoichiometry18m
- Intermolecular Forces (Simplified)19m
- Intermolecular Forces and Physical Properties11m
- Atomic, Ionic and Molecular Solids10m
- Heating and Cooling Curves30m
- 9. Solutions4h 10m
- Solutions6m
- Solubility and Intermolecular Forces18m
- Solutions: Mass Percent6m
- Percent Concentrations10m
- Molarity18m
- Osmolarity15m
- Parts per Million (ppm)13m
- Solubility: Temperature Effect8m
- Intro to Henry's Law4m
- Henry's Law Calculations12m
- Dilutions12m
- Solution Stoichiometry14m
- Electrolytes (Simplified)13m
- Equivalents11m
- Molality15m
- The Colligative Properties15m
- Boiling Point Elevation16m
- Freezing Point Depression9m
- Osmosis16m
- Osmotic Pressure9m
- 10. Acids and Bases3h 29m
- Acid-Base Introduction11m
- Arrhenius Acid and Base6m
- Bronsted Lowry Acid and Base18m
- Acid and Base Strength17m
- Ka and Kb12m
- The pH Scale19m
- Auto-Ionization9m
- pH of Strong Acids and Bases9m
- Acid-Base Equivalents14m
- Acid-Base Reactions7m
- Gas Evolution Equations (Simplified)6m
- Ionic Salts (Simplified)23m
- Buffers25m
- Henderson-Hasselbalch Equation16m
- Strong Acid Strong Base Titrations (Simplified)10m
- 11. Nuclear Chemistry56m
- BONUS: Lab Techniques and Procedures1h 38m
- BONUS: Mathematical Operations and Functions47m
- 12. Introduction to Organic Chemistry1h 34m
- 13. Alkenes, Alkynes, and Aromatic Compounds2h 12m
- 14. Compounds with Oxygen or Sulfur1h 6m
- 15. Aldehydes and Ketones1h 1m
- 16. Carboxylic Acids and Their Derivatives1h 11m
- 17. Amines38m
- 18. Amino Acids and Proteins1h 51m
- 19. Enzymes1h 37m
- 20. Carbohydrates1h 46m
- Intro to Carbohydrates4m
- Classification of Carbohydrates4m
- Fischer Projections4m
- Enantiomers vs Diastereomers8m
- D vs L Enantiomers8m
- Cyclic Hemiacetals8m
- Intro to Haworth Projections4m
- Cyclic Structures of Monosaccharides11m
- Mutarotation4m
- Reduction of Monosaccharides10m
- Oxidation of Monosaccharides7m
- Glycosidic Linkage14m
- Disaccharides7m
- Polysaccharides7m
- 21. The Generation of Biochemical Energy2h 8m
- 22. Carbohydrate Metabolism2h 22m
- 23. Lipids2h 26m
- Intro to Lipids6m
- Fatty Acids25m
- Physical Properties of Fatty Acids6m
- Waxes4m
- Triacylglycerols12m
- Triacylglycerol Reactions: Hydrogenation8m
- Triacylglycerol Reactions: Hydrolysis13m
- Triacylglycerol Reactions: Oxidation7m
- Glycerophospholipids15m
- Sphingomyelins13m
- Steroids15m
- Cell Membranes7m
- Membrane Transport10m
- 24. Lipid Metabolism1h 45m
- 25. Protein and Amino Acid Metabolism1h 37m
- 26. Nucleic Acids and Protein Synthesis2h 54m
- Intro to Nucleic Acids4m
- Nitrogenous Bases16m
- Nucleoside and Nucleotide Formation9m
- Naming Nucleosides and Nucleotides13m
- Phosphodiester Bond Formation7m
- Primary Structure of Nucleic Acids11m
- Base Pairing10m
- DNA Double Helix6m
- Intro to DNA Replication20m
- Steps of DNA Replication11m
- Types of RNA10m
- Overview of Protein Synthesis4m
- Transcription: mRNA Synthesis9m
- Processing of pre-mRNA5m
- The Genetic Code6m
- Introduction to Translation7m
- Translation: Protein Synthesis18m
Experimental Error: Videos & Practice Problems
Experimental error is categorized into random and systematic errors. Random errors indicate a lack of precision, leading to unpredictable fluctuations in measurements, while systematic errors reflect a lack of accuracy, consistently skewing results in one direction. To minimize random errors, multiple measurements should be averaged. The percent error formula, given by , helps assess measurement precision. Understanding these errors is crucial for accurate scientific experimentation.
Experimental error is the difference between measurements, which deviates from a true value.
Experimental Error
Error can be grouped into 2 major categories: Random error and Systematic error.
Experimental Error
Video transcript
Calculating percent error allows a chemist to determine the amount of precision in their calculations.
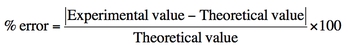
Random Errors
Video transcript
Which of the following features are indicative of random errors? So the first option, doing numerous measurements and taking the average to minimize any errors. Remember we talked about this up above. Random errors are unpredictable. On the same scale that you're measuring something, you may get a total that's 1 gram too high, and then another instance it may give you a measurement that's 2 grams too light. It's always fluctuating between being too much or too low. Here, a way to minimize random error is to take numerous measurements and then take the overall average of those measurements. So yes, this is indicative of a random error. So we know that option 1 is at least true.
The results of an experiment are consistently greater than expected or less than expected. Here it's saying consistently. Consistently would imply that it's a predictable outcome. So this would indicate that we have a systematic error. Remember, systematic errors will consistently give us a value that's greater than expected or less than expected. Never both. So, this would not be an option.
Refining the parameters of the experiments helps eliminate any errors. Now remember, a random error is unpredictable. So there's nothing really we can do ourselves to stop it from happening. All we can do is help to minimize random error by taking several measurements. Here, if we're going to redefine or refine the parameters, that means that we're dealing with a systematic error. One reason that systematic errors occur is that the design of the experiment may be flawed. So we go back and we tweak it a bit to make it better. This helps to eliminate systematic errors.
Finally, the existence of the error is hard to determine. Here it's easy to see that we have a random error. You measure something several times, and you get numbers that are too high and too low. It's always fluctuating back and forth between being too high or too low. We know that's not normal, so we know there's a problem there. A systematic error, though, will consistently give us a number that's too high or give us a number that's too low. Because it's consistently doing this, it's hard for us to determine if an error does occur or not. So this would be indicative of a systematic error. So, out of my four options, only option 1 represents random error.
Now that we've done this example, try to do the final one. See if you get the same answer that I get when you come back and take a look at my explanation.
Systematic Error
Video transcript
Which of the following represent a systematic error when measuring the mass of an anhydrous object? Anhydrous means that the object has been completely dried out. All the water has been left out of the object or evaporated out of the object. Now when it comes to a systematic error, it's predictable in the sense that it's always going to give us a value that's too high or too low from the agreed upon value. But in its predictability, there is some problem involved. Because of this predictability, if I have the agreed upon value ahead of time, which is called your theoretical value, I cannot know for sure if a systematic error is occurring without that value. A good thing about systematic error is if you know that you have it, all you have to do is correct the design of your experiment. By correcting it, you can eliminate that error. Knowing this now, let's take a look at the options:
- You weigh the object before all the water has evaporated. So you're weighing an object, it's supposed to be completely dry, but you haven't given enough time to dry out. So you're going to get a mass that's too high. This is a flaw within the experiment. You didn't wait long enough for you to dry out all of the water. So this is definitely a systematic error. All you have to do to correct it is wait more time. Always wait as long as possible in terms of drying. Get all the water out, and then you'll get the right mass of the dry object.
- The scale used has not been properly calibrated. So this is a big thing. Anytime you start a lab, they always tell you to calibrate your pipettes, calibrate your burettes, calibrate your scales. This ensures that the number that you're going to get is as close to the agreed upon value as possible. So again, that's another flaw within the system. All you have to do to get rid of that error is calibrate the scale.
- Airflow near the balance causes the precise mass to vary. Airflow is hard to control as you're opening up the scale and putting your object inside and then closing the door behind it. So airflow is something that's outside your realm of control. Because of this, it's a random error. That airflow won't always be present so it won't always affect the mass that you have.
- You write down the incorrect mass of the anhydrous object. So here, you didn't write down the correct number. This is a little bit tricky. Could this be a systematic error? Could this just be a random error on your part? Because you're not always going to write down the wrong number, we're going to say here that this could fall under a systematic error because within the design of your experiment, you're supposed to always double-check, triple-check all your numbers and values to make sure they make sense. The fact that you wrote down the wrong number and didn't go back and check it is a flaw in the design of your experiment. You tell yourself, if I write down a number, I have to look at it multiple times to make sure it's the correct number that I'm writing. So we could say that the 4th option would fall under systematic error as well. So here we'd say that 1, 2, and 4 are your systematic errors, although 4, you could be open to saying that it's a random error as well because you're not always going to make that mistake. So, guys, remember the difference between systematic
Do you want more practice?
Here’s what students ask on this topic:
What is the difference between random error and systematic error in experimental measurements?
Random errors are unpredictable fluctuations in measurements, often due to uncontrollable variables, leading to a lack of precision. They can cause results to be either too high or too low. To minimize random errors, multiple measurements should be taken and averaged. Systematic errors, on the other hand, are consistent deviations from the true value, caused by flaws in the experimental setup or equipment, leading to a lack of accuracy. These errors consistently skew results in one direction, either too high or too low. Identifying and correcting the source of systematic errors, such as recalibrating equipment, can help eliminate them.
 Created using AI
Created using AIHow can you minimize random errors in an experiment?
To minimize random errors, take multiple measurements and calculate the average. This approach helps to balance out the unpredictable fluctuations that characterize random errors. Additionally, ensuring a controlled environment and using precise instruments can further reduce the impact of random errors. However, since random errors are inherently unpredictable, completely eliminating them is challenging. Averaging multiple measurements remains the most effective strategy for minimizing their effect on experimental results.
 Created using AI
Created using AIWhat is the formula for calculating percent error, and how is it used?
The formula for calculating percent error is:
This formula helps assess the precision of your measurements by comparing the experimental value to the theoretical value. The absolute difference between these values is divided by the theoretical value and then multiplied by 100 to express the error as a percentage. A lower percent error indicates higher precision, while a higher percent error suggests less precise measurements.
 Created using AI
Created using AIWhat are some common sources of systematic error in experiments?
Common sources of systematic error include improperly calibrated equipment, flawed experimental design, and consistent environmental factors. For example, a scale that is not calibrated correctly will consistently give incorrect measurements. Similarly, if an experiment is designed in a way that introduces bias, such as not allowing enough time for a substance to dry completely, it will lead to systematic errors. Identifying and correcting these sources, such as recalibrating equipment and refining experimental procedures, can help eliminate systematic errors.
 Created using AI
Created using AIWhy is it important to distinguish between random and systematic errors in scientific experiments?
Distinguishing between random and systematic errors is crucial for accurate scientific experimentation. Random errors affect the precision of measurements and can be minimized by averaging multiple measurements. Systematic errors, however, affect the accuracy and consistently skew results in one direction. Identifying the type of error helps in applying the correct method to minimize or eliminate it, ensuring the reliability and validity of experimental results. Understanding these errors also aids in interpreting data correctly and improving experimental design.
 Created using AI
Created using AI