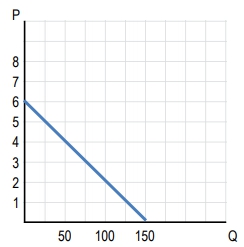
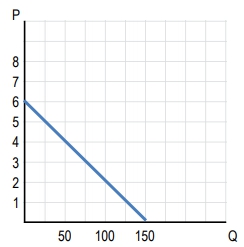
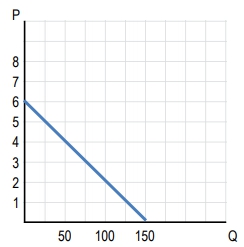
So now let's see how elasticity changes along a linear demand curve. A lot of students mix this up and tend to think that slope and elasticity are the same thing. Although they are slightly related, they are not the same, right? Slope is a ratio of changes between two variables where we're in a certain unit, but elasticity is a ratio of percentage changes, right? We've got percentage changes, and that little word "percentages" there makes a huge difference between the two definitions. So let's go over to this purple box where I can maybe show you an example of what's happening. When we have a price of $1 and we increase it to $2, what was our unit change in that situation? Well, we went from 1 to 2; we increased by 1, right? We increased by $1 there. But what was our percentage change? We started at 1 - whoa, calm down. Okay, we started at 1, and now we're at 2. We doubled our value, right? We started at 1 and doubled it up to 2. So our percentage change in that situation was 100%. We increased it 100% from 1 to 2. And how about this second situation? We were at a price of 2, and now we increased it to 3. Again, we have a unit change of 1, right? We just increased it by $1 there, but what about our percentage change this time? It's not 100 percent, right? We didn't double it again. We actually only increased it by half of the amount. We went from 2 to 3, we only increased it by 1, which is half of 2. In that situation, our percentage change was just 50% right, and that's because the numbers got bigger, right? So you could imagine from 3 to 4; we're still going to have a unit change of 1 but a smaller percentage change. 4 to 5, the percentage change is even smaller. So you're going to see that even though the change is linear, right, we have this constant change of 1 unit at a time, we're having different percentage changes every time, right? So even if you don't get everything on a really deep level there, just at least understand that we do have a difference here between slope and elasticity. Cool? So let's go ahead and go on to this graph where we've got a linear demand curve, and I just want to cut to the chase real quick. When we've got a linear demand curve, we're going to have sections of the line that are elastic, sections of the line that are inelastic, and a point on the line that's going to be unit elastic, alright? And that unit elastic point is going to be the point where we want to produce, and it's the point where revenue is maximized, okay? So I'm just going to cut through all the crap here and go straight to it. So right here, this section here to the left of the middle, right? So when you connect your demand curve from one axis to the other, like we have here, it's touching the price axis, and it's touching the quantity axis; you just go to the middle point, right? Right here is the middle, and you can visualize that very easily. Where's the middle of that line? Right there. So to the left of that middle point, which in the middle I'm going to highlight in green, right, to the left of that middle point, we are going to have elastic demand, and to the right of that point. What do you guys think? Yep. I heard someone. It's inelastic on that side of the point. Now here's the real kicker. Do you guys think at that specific point, what are we dealing with? Yep. Unit elastic is at that point right in the middle, and at that unit elastic point, just like I said, that's where we wanna produce, and that's where revenue is maximized. So let's go ahead and go to this table right here, where we've got the prices and quantities demanded, which are shown on that line on the graph. Okay? So I've gone ahead and taken these points and plotted them on the graph right there. Let's go ahead and calculate our total revenue in each of these cases. So total revenue, remember, is just price times quantity. So all we got to do is just multiply across here. So price times quantity, 0. 7 times 2, 14. It looks like we're doing our multiplication tables here again, so you could journey back to arithmetic, but let's go ahead and fill out this table. 34 times 8. 32. Right. So I'm just multiplying across price times quantity, and there we go. We've got all our total revenues. So you'll notice what's happening, right? We started with a revenue of 0 when we had a price of 8 and no quantity demanded. And as we lowered the price and people started to demand quanti...
4. Elasticity
Total Revenue Along a Linear Demand Curve
4. Elasticity
Total Revenue Along a Linear Demand Curve - Online Tutor, Practice Problems & Exam Prep
Elasticity changes along a linear demand curve!
1
concept
Total Revenue Along a Linear Demand Curve
Video duration:
7mPlay a video:
Video transcript
2
Problem
ProblemWhat is the elasticity of demand when the price of the good changes from $3 to $5?
A
0.25
B
0.50
C
1.00
D
2.00
3
Problem
ProblemAt what price is the elasticity of demand for the product equal to one?
A
$2
B
$3
C
$4
D
$5
4
Problem
ProblemAt what price is revenue maximized?
A
$2
B
$3
C
$4
D
$5