1. Chemical Measurements
Volumetric Titrations
Classical Stoichiometric Methods involve the use of both volumetric methods (titrations) and gravimetric methods.
Titrations
1
example
Volumetric Titration
3m
Play a video:
Was this helpful?
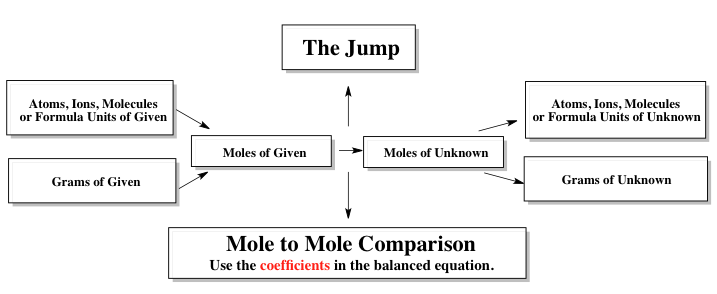
tight rations are an important topic that we're going to discuss when it comes to historical metric reactions. But we'll talk about it consistently throughout many of the chapters, as we delve deeper and deeper into analytical chemistry here, we're gonna say that connected to the idea of tight rations. We have other terms that you should recall here. We're gonna say that when we're dealing with gravimetric analysis, this is just basically helping us to determine the amount of product that we can isolate within a chemical reaction. And we're gonna say the calculations that we use to determine that isolated amount is termed stoic geometry. Now, with stock geometry, we have to employ the use of the story geometric chart. Remember with the story geometric chart will be given a balanced chemical reaction and from the balanced chemical reaction will be given amounts for either some elements and compounds. And asked to find the unknown quantity of some other element or compound within that balanced equation. Now, with the psychometric chart, we have are given information which typically is given to us in some units of mass or substance. So they may give us given amounts in grams, um possibly moles. Remember when we're going from grams to moles, we use the molecular weight of the compound itself. Um they may give us the initial amount in atoms ions, molecules or formula units. In this case to go to moles. We'd use avocados number Which is 6.02, 2 times 10 to the 23. Remember when we use the term atoms atoms is for basically neutral single element. So if we're talking about oxygen adam, we're just talking about oh we use the term for ions. When we have a single element with the charge, then the term molecules is used for Kovalev compounds, which is just non metals connected together. And formula units is used for ionic compounds where we have a positive ion connected to a negative ion. Now using the Stark geometric chart will be given information. I'm usually one of these values and it's our job to go to the unknown amount of our other compound or element within the balanced equation. Now when we're looking for the unknown, they may ask us to find the unknown in moles, Adams, ions, molecules, formula units, or grams itself. And remember as we're going from an area where we know stuff because it's given to us to an area where we know nothing at all. We have to make basically a leap from one side to the other side. That's why we call this the jump as we go from the side where we are giving information to the side where we have our unknown. And remember when we make this jump, we have to do a multiple comparison. That's why a balanced equation is needed with geometry. Because when we do this multiple comparison, we have to use the coefficients within the balanced equation. Knowing this basic set up for the story geometric chart will be essential for any type of titrate in Stark geometric calculations that we're gonna employ. Now that we've looked at that chart, come back, click on the following video and take a look at how I answer the following example, question that's left on the bottom by using the geometric chart and what we remember dealing with story.
2
example
Volumetric Titration
8m
Play a video:
Was this helpful?
So let's take a look at the example given below here, it says that iron three can be oxidized by an acidic potassium di chrome eight solution according to the net ionic equation below. Were asked how many micro leaders of a 80.250 moller iron to chloride solution are needed to completely react with a 9.12 g of a compound containing 41.5% potassium di chrome eight. Alright, remember with any type of word problem. Even so geometric ones we always start out with, What exactly are they asking me to find? We're being asked to find micro leaders micro leaders of my iron to chloride solution. Next we write down all the given information that we have. So from the information that we can see we have 0.250 moller iron to chloride. What is that really saying? Remember polarity is moles over liters. So that's really saying that we have 0.250 moles of iron to chloride over one leader we have 9.12g of a compound and it's saying that of that 9.2 g of that compound, 41.5 weight percent of it is potassium di chrome eight. That would mean that this weight percent. Here is really saying that we have 41.5 g of potassium di chrome eight for every 100 g of the compound itself. Now remember what we're being asked to find can always be located in some way within the given information here. We want micro leaders of our iron three chloride solution and we need to realize that we have leaders of my iron to chloride solution there. So we know that we need to end with that polarity in some way. Right now we're gonna start out with a 9.12 g because that's just one unit by itself, easier to manipulate. We're gonna bring down The 9.12 g of my compound. We want this compound to cancel out. Always important to write three units so you can see which way to head. The only other place that has compound involved is right here. So we know we're gonna use next, the weight percent, bring down the 100 g of the compound and then we have 41.5 g of potassium bromate on top. Next. Since we have a balanced equation, we're gonna have to form a stoke geometric relationship with potassium diet Crow mate. And connected in some way to iron to chloride. To be able to establish the stoke geometric relationship we're gonna have to convert right now are potassium diet Crow mate from grams to moles. So we have one mole of potassium di chrome eight on top and then we have the grams of potassium di chrome eight on the bottom. potassium di chrome eight itself has involved to potassium to chromium seven oxygen's. Remember analytical chemistry is chemistry of precision. So you have to get the full value of their atomic mass is found on your periodic table. Okay, so no rounding to the very end. So here, according to our periodic table, potassium weighs 39.983 g chromium is 51.9961 g, oxygen is 15.9994 g. Multiply those things together. Okay, and then you're just gonna add up all three of these masses together. When we do that, we get to 94.184. So g cancel out. And now we have moles of potassium di chrome eight, which is great. But we run into another issue right now. What we have is moles of potassium micro mate. But if we look at our balanced equation, potassium chromium is found nowhere. But we have though is we have chrome eight involved. So what we're gonna do next is we're gonna convert the moles of my potassium die chrome eight for every one mole of potassium di chrome eight, we have exactly one. We have exactly one di chrome eight. So we have one mole of di chrome it within that formula. So right now we have our molds of di chromite which we wanted. And the reason we need to do this is because next we're going to say we need to establish a relationship um that allows us to get to information on iron to chloride. Mhm. If we take a look here, iron to chloride is made up of iron too. It's made up of iron too. And chloride ions realize here that iron to chloride has in it, this particular ion which is a good thing because it has that ion in it. We can establish a stoke geometric relationship between F E two plus and die chrome eight. We can say according to my balanced equation for every one mole of micro mate we have six moles of iron too because I have the mold of iron to Now I can next make the jump to moles of iron to chloride. So we're gonna say here for every one mole of the entire iron to chloride compound I have in it. Exactly one mole of iron too. Okay, so this problem is pretty long. So make sure you're following along every step of the way on how we're canceling out units to isolate what we want at the end. Now that I have moles of iron tube, I can use the polarity From earlier. So we put the moles of iron to Clyde on the bottom. So .250 moles of iron to chloride on the bottom And then one leader on top. So we're gonna continue down here. We have leaders here on the top. Right, so we want to get rid of them. So we put leaders on the bottom and micro leaders on top. Remember metric prefix conversions for everyone? Micro it's 10 to the negative six. So at the end we're gonna have our micro leaders of our solution based on the numbers that we see. This has three sig figs in it. 36 figs in it and 36 figs in it. So we want to answer at the end to have three sig figs as well. Okay, so that's gonna be 3.09 times 10 to the five micro leaders as my final answer. So just remember that when it comes to stoke geometric calculations or tight rations, we have to follow a psychometric chart at times in order to isolate the desired units that we want at the end. So make sure you remember the ins and outs of the stoking metric chart and how best to use it to use the same approach is we've done earlier in terms of dimensional analysis solving, write down what they're asking you to find. And then on the other side write down all the given information. What you're being asked to find can always be located somewhere within the given information. You just have to look closely enough
Titrations Calculations
3
example
Titrations Calculations 1
5m
Play a video:
Was this helpful?
we continue our discussion of tight rations with the following example question here, it says magnesium reacts with hydrochloric acid according to the reaction below. Were asked how many grams of 5.310% by weight of acquis magnesium are required to provide a 25% access to react with 75 mls of 750.550 molar hydrochloric acid. So in this question, what they're asking us to find is the grams of my acquis magnesium solution. So we're looking for grams of solution from the information provided we need to isolate that variable. So if we take a look here, we're gonna say That they're giving us 5.310%. So what does that really mean? That means that we have 5.310 g of magnesium for every 100 g of solution. We're told here that we have 75 mls of 750.550 molar hydrochloric acid. Remember the word of means multiply. So, we need to realize here that if I can change my mls into leaders and then multiply it by the polarity, I'll get the moles of hydrochloric acid. So that's what we're gonna do. We're gonna change mls into the leaders. Remember one leader is 1000 mL. That polarity. There really means 10000.550 moles Of hydrochloric acid over one liter of solution. And now that we have the moles of hcl realize here That's gonna give me .004125 moles of hydrochloric acid. This number here represents 100 Amount of hydrochloric acid. But in the question itself, it's saying that we need to do a 25% excess. So that means that we're gonna take these moles and we're gonna multiply them by 25% access. So that's 100% plus 25%. Which is 125%. Again, we can't use percentages within our calculations. So we'll have to divide this by 100. So that's 1.25. When I multiply these moles by 1.25, that gives me a new set of moles as .005156 moles of Hcl. So this represents my 25% excess. Alright, so now all we have to do is find a way to get two g of solution. And we're gonna realize that to get to their here's our grams of solution. I'm gonna need to cancel out these grams of magnesium. So at this point we're gonna use store geometry to get two g of magnesium so they can cancel out with these grams of magnesium. So at the end we're left with grams of solution. Alright, So what I'm gonna do here is I'm gonna try to get rid of moles of hydrochloric acid. I need to find moles of magnesium here, we're gonna do a multiple comparison and say for every one mole of magnesium, I have two moles of hydrochloric acid. So moles cancel out Next, we're gonna use the periodic table and say according to my periodic table for every one mole of magnesium, the weight is 24.3050 g of magnesium. Now, finally, because I have grams of magnesium, I can now bring in this weight percent down. So 5.310g of magnesium on the bottom, 100 g of solution on top. So that equals 1.18006 g of solution. And here we have four significant figures in this value to significant um significant figures. Three significant figures. And we have here Three significant figures. So at the end of this, our answer is gonna have two significant figures which comes out to 1.2 g of solution so that that represents the grams of our solution. Realize that this is just a typical type of tie Trish in question. Similar to what we saw on the previous page. When we dealt with the example, just remember to first write down what you are being asked to find. Then write all the given information. What you're asked to find can always be located in some way within the given information.
4
example
Titrations Calculations 1
4m
Play a video:
Was this helpful?
so continue with our discussion of volumetric penetrations. We have example to hear it states the density of 2.20 molar solution of methanol is 0.976 g per mole leader here it asks us what is the morality of the solution? The molar mass of methanol is given as 31.34 g per mole. Now remember that morality is lower case m Now morality equals our moles of solute divided by kilograms of solvent. Now this is gonna require a bit of work on our part in order to isolate moles of solute and the kg of solvent from the information given we see that we have 2.20 molar of solution. Now remember polarity itself equals moles of solute over leaders of solution. So there's 2.20 moller is equivalent to 2.20 moles I saw you which would be methanol Divided by one L of solution. Also were given the density of our solution. So the density of our solution which is D is .976g of solution per one middle liter of solution. The molar mass is given information as well but we can calculate that on our own. So you don't even need to consider that given information. At this point. Now without even doing any math, we can see that we can isolate the moles of solute because for polarity we have the moles of our salute which is methanol. So we already have that part figured out. Now the hard part comes from identifying your kilograms of solvent in order to do that, realize that we have the volume of our solution which is one leader And we have the density of our solution. We're gonna take those one liters of solution and say that they are equivalent to 1000 ml of solution. Then I'm gonna bring in the density So one ml of solution here on the bottom. And then we have .976 g of solution on top. When we multiply those together, that's going to give me 976 g of solution. Now remember a solution is comprised of two parts. It's made up of a solute and a solvent together. That means if I can isolate out the saw you. But what I have left is grams of solvent. Remember our salute is methanol. We have the moles of methanol from the very beginning. I'm gonna convert those into grams and subtract them for the from the grams of solution. And what will be left will be our grams of solvent. So take the 2.20 moles of methanol. Use the molar mass given to us. So it's one mole of methanol For every 31.034 g of methanol. When we do that, that's gonna give me a mass of 68 .2748 g of methanol. Okay, let me take myself out of the image for 1/2. So that's our massive salute. Subtracting from the 976 will give us our grants of solvent. So when we do that, we're gonna get 907.7252 g of solvent. Now that we have those grams of solvent just changed them into kg. So we have 907.725g of solvent. And we're gonna stay here for every one kg. There's 1000 g. That's 10000.9077 to 5 kg of my solvent. Take that and plug it up here. So when we divide those two numbers are gonna get 2.42 molo as our answer. So that will represent the morality of our solution. So remember we can isolate morality of a solution if we have the density of the solution and the polarity of the solution, use those approaches. You'll be able to answer any questions similar to this one.
5
example
Titrations Calculations 2
7m
Play a video:
Was this helpful?
Yeah. In this video we take a look at a backed immigration. Now in a back to trey shin. We have two compounds reacting with one another. And at the end we're gonna have an excess remaining of one of the re agents with that access. We then reacted with a second compound. This can help us determine the concentration of the initial an elite or can help us to figure out volume of the second compound being used. The variety of questions from a back to trey shin can be endless depending on how you write it. So, in this first example, we'll take a look at a typical type of backed iteration question. So here it says 0.4317 g sample of calcium carbonate is added to a flash that also contained 12.50 mls of 1.530 Moeller hydro Tomic acid. Now here we have our balanced equation for hydrochloric acid. It says additional water is then added to create a 250 ml of solution. A next 20 mls a liquid solution of a is taken and tight traded with 200.980 molar sodium hydroxide were asked at the end how many milliliters of sodium hydroxide were used. All right. So in this question, we need to figure out what our mls of sodium hydroxide are. And what we need to realize here is that we have potassium carbonate reacting with hydra bronek acid. Mhm. And we're gonna say here if we were the first step we're gonna do is just set up a regular store geometric example. We're gonna say here that we have .4317g of calcium carbonate. We're gonna stay here for every one mole of calcium carbonate. How many grams of calcium carbonate do we have in that? Well we say that calcium we have one calcium one carbon three oxygen's Based on the periodic table. Their masses are 40.078g, 12.0107 g And 15.9994 g. Multiply across add up the totals gives us 100.087 g of calcium carbonate. Mhm. At this point we're now going to see what this Tokyo metric relationship is between calcium carbonate and hydroponic acid. So here we're gonna say according to my balanced equation for every one mole of calcium carbonate, there are two moles of hydrochloric acid. Yeah, all we're doing at this point is just figuring out stoking stoking metric li how many moles of hydrochloric acid would be needed to completely react with this amount of calcium carbonate. That's all we're doing. So for that many grams of calcium carbonate, we need exactly 0.0.86 to seven moles of hydroponic acid. What we have to do now is we have to figure out from this volume in this polarity. How many moles of H. B. R do we actually have present. So we're gonna change our mls in two leaders. Remember your polarity as moles over leaders. Mhm. So that comes out to .019125 moles of hydra bronek acid present. So you can clearly see that the amount of HBR needed is smaller than the amount of HP are that we have present. So that really ask us now how much of the HBR was was left unused. So we have this initial amount of hydro bronek acid minus what we need. So this is how much hydroponic acid we have left from the first equation. So this is how much how many moles of H. B. R we have left And we're told that water is added to this moles of H B. R left until we have a volume of 250 mls. So that's saying that we have .01048 moles of HBR and it's connected to .250 L. All I did here is I converted mls into leaders. Next they say that we take 20 ml of that amount. Okay, so all I'm doing is I'm taking 20 mls of that hBR So what does that mean? So 28 miles when I change it to leaders is .020 L. That portion is just telling me to multiply those two together to figure out how many moles of H. B. R. I took out from just that 20 ml a liquid portion. And remember we're trying to figure out how many mls of N A. O. H. We have, we have the polarity of N. A. O. H. So what we're gonna do here is we're gonna say according to this second equation for every one mole of N. A. O. H. I have one mole of H. B. R. So for every one mole of H. P. R. We have one mole of N. A. O. H. Then all I have to do at this point is I'm gonna use the polarity now, that's right here Which is .0980 moles of N. A. O. H. For everyone leader. And for every one leader we have 1000 middle leaders. So at the end that gives me 8.5698 MLS. And here we're going to do 36 fix because this number here has three sick fix compared to the others which have four. So this is 8.57 mls of N. A. O. H. Would have been needed in order to completely neutralize any excess HBR left. So this is a little bit different from more customers seeing with iteration questions remember in a back titrate in question. Um We utilize two different equations will have some excess of an analyst left. So we'll have to use a second compound in order to neutralize the remainder of that an elite doing that can help us to determine the polarity of the second compound, its volume, even its molar mass. In this example we use that excess HBR and and compared it to the n a o. H in order to figure out the volume of the n a o h that was present.
6
example
Titrations Calculations 2
8m
Play a video:
Was this helpful?
a 9.2476 g sample of M O H two was mixed with 15 ml of 1.530 moller hydro biotic acid and diluted to a final 125 ml of solution, he were told that a 12 ml alec wad of this diluted solution was taken and hydrated with 18.23 mls of 0.695 Mueller and a O. H. Were asked what is the identity of the metal representing? Em All right, so this again is a continuation of our discussion of backed it rations. In this case, we need to determine what the identity of R. M. Variable is. Which means that we need to determine its molecular mass or its molar mass. And to be able to do that, we need to realize here that molecular weight or molar mass equals grams per mole. And what we're gonna have to do here is determine what the molar mass of the entire Moh- two compound is. And from that subtract out the amount of oxygen and hydrogen and the remaining amount will be the mass of that unknown metal. At the moment we know that the mass of this unknown compound is this So we have the grams figure it out. All we have to do is determine how many moles of this compound did we have originally from all the information given. So let's break down and dissect the portions that they're giving us to get that last piece that we need. So they tell us that at the end of this process we have an excess of our hydro ionic acid remaining and that access that we had required this Ml and this polarity of N. A. O. H. In order to neutralize it. So what I'm gonna do here is I'm gonna change those Mls into leaders and then I'm gonna multiply it by the polarity. Remember polarity really means moles over leaders. Yeah. And we're gonna say here that the relationship between sodium hydroxide and the acid itself is a 1-1 relationship offering one mole of N. A. O. H. I have one mole of H. I. This will tell me how much H I had left as excess after it reacted with this particular base here. So at this point I know at the end that I had this many moles of hydro ionic acid left next. What I'm gonna do is I'm gonna determine how many moles of hydro biotic acid I had from the very beginning in the very beginning I had this volume and this polarity of hydro biotic acid. So change those Mls into leaders. So at 1.530 moles of hydrochloric acid per one liter. Yeah So that means we had .02295 moles of hydro biotic acid. Originally by subtracting those two. That'll tell me how many moles of hydro biotic acid reacted and with that information I can figure out how many moles of this I had. So I'm gonna take those two moles that I had and subtract them from one another. So the moles I had originally minus the moles that I had left as excess. And this is how many moles reacted. Mhm. Because of that. Now I can do uh basically uh comparison between the two, We're gonna say here that we have .021683 moles of H. I. And according to my equation, for every two moles of H. I, we had one mole of this unknown compound. So here we have .010842 moles Of M. O. H. two. But remember, it's not as simple as this because we do have a dilution occurring where we're diluting it 225 ml of this h. I solution and we're taking a look watts of it. So those play a role in determining the true number of moles of M. OH two. So, if I want to find the true number of moles of M. O H two within the 125 ml solution. This is what I do. I'm gonna take those moles that I just found. We're gonna say that we had 12 ml, a lot of my acid and I've already converted the moles of acid into moles of base. So now I can just combine those moles of base with the 12 mls of Allah Remember the dilution at the end was 125 MLS, which is .125 L. Doing this allows me to find the true moles that I have of this unknown compound. So there goes my moles of that base. Remember molecular mass or molar masses grams per mole. So take those and plug them here on the bottom. So here we'll have the molar mass or molecular weight of the compound as being 81.88-1 g per mole. With this information, I'll be able to find the identity of my metal. M realize that we already know that it contains oxygen and hydrogen in the formula. There are two oxygen's and two hydrogen within this formula. So take their atomic masses from the periodic table. The both um the combined weight of the two oxygen's and the two hydrogen comes out to 34.147 g per mole. Um yeah grams per mole. So take those 34 and change of g per mole and subtracted from the total molecular mass that we found. When we do that, we'll get 47.867 g per mole. And all we have to do at this point is look at the periodic table and see which metal has a close enough mass to that number. If we look, we'll see that the closest element is titanium. So M represents titanium. So the compound is titanium to hydroxide. So we continue with our discussion of backed it rations. And with this information, in this case, we're able to determine the molar mass of a particular element based on the information given, remember determining the amount of excess of your compound at the end is essential for determining the amount of it that has reacted by knowing that we can work backwards in order to figure out the in this case molar mass of the base used in the first equation.
7
example
Titrations Calculations
9m
Play a video:
Was this helpful?
here. It says a 6.2034 g sample of copper O. H. We don't know how many hydroxide we have was mixed with 25 ml of 2.250 molar hydrochloric acid and diluted to a final 250 ml of solution. We're told that a 50 ml of this solution was taken and I traded with 16.25 mls of 0.1250 moller potassium hydroxide. Were asked what is the value of N. All right. So in this question, we don't know what our end values are here and here and here, based on what we've learned thus far dealing with backed it rations. We'll have to utilize some of the techniques we've seen previously. In order to answer this particular and unique question, what we're gonna do first is realize that they're giving me the amount of base that was used to neutralize the excess hydro biotic acid that we had. So we're gonna use this volume and polarity of K. O. H. In order to figure out how much H. I had left as excess. So we're gonna take the 16.25 mls and change that into leaders. And then you're gonna multiply it by the polarity to figure out the moles of K. O. H. And remember based on the second equation for every one mole of K. O. H. There's one mole of h I. So it's a 1 to 1 relationship. So this here represents the moles of hydrostatic hydrostatic acid that we had left is access. Next, what I'm going to do here is I'm going to determine how much hydrochloric acid I had in the very beginning. So change those mls into leaders And then multiply it by the Polarity, which is just simply moles over L. So that gives me .05625 moles of hydro biotic acid that we had originally. Now realize here, at this point, we can subtract those two numbers and that'll tell me how much hydro acetic acid must have reacted. So take the two totals that we have here, Subtract them from one another. That's gonna give me .054219 moles of hydro biotic acid left. So I'm gonna bring this down now realize we have these moles of hydrochloric acid, they were diluted to 250 mls of solution And we took 50 mls of it. Now with a dilution equation, remember we used to say M one V one equals M two V two. In this case we're not gonna worry about polarity but we're gonna focus on the moles. So you can think of it like this. We have these moles here. They are diluted to 250 mls. So if we change that to leaders, that's 2500.250 liters. We don't know what our second set of moles will be. But we do know that we took 50 mls, Which comes out to .050 L. So divide both sides by .050 L. Okay, and that'll tell me how many moles of hydrochloric acid are involved. Mhm. So that'll give me .271095 moles of hydro ionic acid. After the dilution have been taken into account. Now at this point, remember we're trying to figure out what end represents. So since we know the moles of hydrogen ionic acid, I'm gonna say here, according to my balanced equation for every n moles of H I I'm gonna have one mole of C. U. O H. N. Okay. And remember, that's the coefficient here, it's n. This is gonna be important to help us figure out what the answer will be. Now we're gonna say at this point, that formula mass equals your molar mass. Okay. Of this compound times the number of moles of that compound. And we're gonna do is we're gonna start plugging in the information that we know. The formula mass is just the amount of that substance that were given. Were told that we have 6.2034g of it. So we're gonna plug that in Here. We know the molar mass of copper involved. Copper on our periodic table, weighs 63.546 g. We know that we have oxygen and hydrogen. I just don't know how many of each I have. So we're gonna say that we know we have the mass of oxygen and hydrogen which comes out to 17.0073g when you add them together. I just don't know what that number is getting multiplied by. So I keep the end around times now the moles of the compound come from this portion right here because the moles will cancel out. And what I'll have left is moles of the compound divided by N. So the moles of my compound is .271095, divided by N. With this I can try to solve for end now. So we're gonna take this portion here and distribute it into here and distribute it into here. So when I do that I'm gonna get 6.2034g equals so when I distribute at 63.546 times .271095 divided by N. Plus. Now these two are multiplying each other. So that's 17.0073 n. times .271095 divided by N. Okay, now I'm gonna bring the math over here. So 6.2034 equals when I multiply these two together. That gives me 17.227 divided by N. When I multiply these together, that's gonna give me 0.461059. N. Still divided by N. At this point the ends cancel out. So we have 6.2034 equals 17.227 divided by n plus .461059. So we're almost there. Just gotta subtract this out from both sides and we'll be able to isolate my end variable. So at this point it gives me 5.74234 equals 17.227 divided by N. When we isolate N. N equals 17.227 divided by 5.74234. And at the end that equals three. So that means that the number that's missing here would be three. So that's copper three hydroxide. We have three moles of H I three moles. Um we have three here and three there. So that's the value of my end. So this question is a bit different from what we saw in the previous backed iteration question. But remember, like I said before, there's numerous possibilities that exist when dealing with Bhakti tradition questions. We've seen how to calculate volume, the molar mass and in this case the coefficient involved in our given equations. So from the information that we're able to to use were able to figure out what the coefficient and what the subscript would be for each one of the compounds in the first equation. Now that you've seen all of those try to attempt to do the practice question that's left here on the bottom um utilize what you've learned thus far, whether it be titrate asians or back tight rations to see if you can isolate the correct answer to the practice problem left on the bottom page. If you get stuck, don't worry, just come back. Take a look at how I approach that same exact question. So good luck, guys.
8
Problem
ProblemA 1.000 g sample of Na2CO3 (MW: 105.99 g/mol) is dissolved in enough water to make 200.0 mL of solution. A 25.00 mL aliquot required 32.18 mL of HCl to completely neutralize it. What is the molar concentration of HCl?
Na2CO3 (aq) + 2 HCl (aq) → 2 KCl (aq) + H2O(l) + CO2 (g)
A
0.5864 M
B
0.07330 M
C
0.01832 M
D
0.002359 M