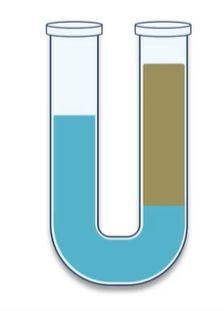
Hey, everyone. So in this problem, we're going to cover a type of pressure gauge used to calculate pressure, called a U-shaped tube or sometimes it's called a U tube. So let's check this out here. This is going to be a pretty long video, but there's some really important information here. So let's check this out. Remember, all pressure gauges use height differences to calculate pressure. And just like always, we're going to use our pressure equation, pbottom=ptop+ρgh. Alright, so this is basically what a U tube shape looks like, or a U-shaped tube literally looks exactly like how it sounds. It's a U-shaped tube that could be open on both sides or could be opened on one side and closed on the other. Alright, let's look at the first couple of cases here, which are relatively straightforward. If you had this open end on both sides, so if you have air basically and it's open over here, then what happens is, if you fill this thing with one liquid, like let's say water or something, it's basically just going to seek to level itself out like this. Because these things are both touching air, they're going to be at the same pressure and they're going to have the same height because it's the same liquid. Right? So that's really straightforward. There's nothing new there. It's actually pretty trivial. The second one is where you have one liquid end where you have a vacuum on one side. The only way you can get a vacuum is where you close off one of the ends like this. So what happens here is, if you were to fill some liquid inside, what happens is, it's going to level off here. But then, over here, what do you think is going to happen? Is the level going to be higher or lower than this point over here? Hopefully, you guys realize it’s actually going to be higher like this. And the reason for that is that if you have air that is pushing down on this with presumably, let's say, standard atmospheric pressure, then basically what happens is if the water level is like this, you're going to push down more with one atmosphere because there's no pressure over here. If you have a vacuum, this pressure is going to be zero. And so therefore, what happens is it's going to sort of lopside like that. Alright? So you're basically just going to have that this column here is going to go up. Alright? So, that's pretty straightforward. Now let's take a look at when things get a little bit interesting. Alright. So these sort of both cases were kind of trivial in the sense that, you know, there's not really a whole lot of new physics going on there. But the most sort of common case in most of your problems is going to involve when you have two liquids and both the sides are open and you're going to dump one liquid into one side. Here's what I mean by this. So let's say you have one liquid over here. I'm going to call this blue like this. Right. And you're going to take another liquid of a different density, so I'm going to have this is going to be ρ1, and I'm going to have another liquid that I'm going to sort of drop in here like this. Right? So I'm going to fill this whole thing up with like a little column like that. Now because this thing is going to be extra weight on the right side, it's going to want to push down and it's going to try to level itself out. Now what's really important here is that it's not going to level out in such a way that it's basically going to get even again because what happens is you now have two different densities. Remember, you're going to have the same liquid at the same height equal to the same pressure, but these two things, if they're different densities, they're not going to have, it's not going to be the same liquid, so they're not going to try to level themselves out. Instead, what's going to happen is that the right column is going to push down just a little bit, and then it's going to find sort of like its new balance like this. So it's going to sit and it's going to rest on top and basically, we're going to have this little rectangle like this. Right? So this is going to be your second liquid on the right side. Now, what happens is you've displaced this amount of water, so that's going to have to appear on the left side. You're going to have to go basically up by the same amounts, roughly over here. And so basically, this is going to be where the new water level is. That's all the water that you've added by displacing from the right side. Now, what happens is there are four really important points in these U-shaped tubes. So I kind of want to go over them really quickly here. The first thing is that you want to call the left side one because that's where all the left liquid is. I'm going to call this one here the second one on the right side. Alright. So there are a couple of important points here. The first is going to be where the top of the left column finally ends up when it's finished. I'm going to call that point A. The second point here is actually going to be the points where if you draw it all the way across, it's basically going to be where the boundary of the two liquids are. So this point right here, I'm going to call this point B. And if you go all the way across, this point here is going to be point D. Alright. And at this point, which is the top of where the right column finally ends up, is going to be point C. Alright. So there are a couple of important distances to sort of note here, which that this distance is going to be. I'm going to call this h1 and this distance here is going to be h2. Alright. So the height of the actual column itself is not going to be equal to the amount of water that it displaces. And again, it's because these things are different densities. Alright. So, what happens here is there's another height, which is going to be basically where the top of the right column is relative to the top of the left column. So it's basically just like the difference in the heights between the right and left columns. Because it's a difference in heights, I'm going to call this Δh like this. And what we can see here from this diagram is that these two things sort of are the same level like this, then that means that Δh just has to be the difference between h2 and h1. So just to put some numbers real quick, imagine this was 10 and imagine that this was 7, then this would have to be Δh3. Right? That's kind of just an important equation. Alright. So in other words, the first equation that we're going to have for these U-shaped tubes is that Δh, the difference in height, is really just going to be the height of the second column minus the height of the first. Right? So it's going to be the difference between the two column heights like this. Now what happens is in some problems, this actually could go lower and you could have the left side go on top. So in order just to make all the units positive in order to get everything positive and not get any weird negative numbers, you just stick an absolute value sign in there."
19. Fluid Mechanics
Pressure Gauge: U-shaped Tube
19. Fluid Mechanics
Pressure Gauge: U-shaped Tube - Online Tutor, Practice Problems & Exam Prep

Get help from an AI Tutor
Ask a question to get started.
1
concept
Pressure Gauges: U-Shaped Tube
Video duration:
18mPlay a video:
Video transcript
2
Problem
ProblemWater and oil are poured into a u-shape tube, as shown below. The column of oil, on the right side, is 25 cm tall, and the distance between the top of the two columns is 9 cm. Calculate the density of the oil.
A
360 kg/m3
B
560 kg/m3
C
640 kg/m3
D
1562 kg/m3