Hey, everyone. So by now, we should be pretty familiar with the idea of Electropotentials and potential differences. In this video, we're gonna cover an idea called equipotential surfaces which are really just these boundaries where you have the same potential. We're gonna see how that relates back to things like electric fields and I'm gonna show you a quick example. Alright? So let's get started. So the word equipotential, right, equi just means equal, potential means potential, basically just means that these are surfaces in which you have constant potential. Alright? So what this means here is that along these surfaces, ΔV=0. So what does that mean? So remember that for a point charge, the potential equation is kqr. Alright. So we just have a constant. We have the charge and basically what this means here is the potential is related to the distance away from the point charge. So if I took this point charge here and if I went a distance I'm gonna call this R and if I did that for a bunch of other locations like this and I would always go out the same exact distance r, then I would just end up with a circle of points like this. Mean, I could just connect them all. So if I went anywhere along this circle, I would measure the exact same potential. I'm just gonna go ahead and put a number to this just to make that a little bit simpler. I'm gonna say this is 10 volts. Alright? This is what an equipotential surface is. It's basically just this surface here in which you're gonna have constant potential as you go all the way around. Okay? And so the potential the equipotential surface for point charges just looks like circles. And by the way so there's a couple of things you should know. The one is that, you know, this is the equipotential surface for 10 volts, but there's actually an infinite number of them. Right? So there's another equipotential surface here and this is gonna be for, let's say, this is, 20 volts. Right? There's gonna be another one all the way out here and this is gonna be for 5 volts or something like that. Right? So there's an infinite number of them each corresponding to a very specific number for that potential. Alright? So, before we move on and draw the potential for a dipole, there's a couple of important rules that you need to know. They have to do with the relationship between the electric field and the potential. So just to sort of recap the equation for the electric field you may have seen, this is gonna be -ΔVΔx. What this just means here is that anytime you have a potential difference like you do along these equipotential surfaces here, So here you're gonna have a ΔV. Right? Because your potential is dropping from 20 to 10 to 5. Then you're gonna have an electric field and the electric field points outwards like this. Alright. So another way you could also see this equation written is ΔV=-eΔx. This is just taking this equation over here and rewriting it for ΔV. Now the one thing that's really important you need to know is that this electric field is always going to be perpendicular. So it's always perpendicular to the equipotential surfaces. So notice here how the electric field lines point outwards radially away from the point charge and the equipotential surfaces are circles so that anywhere along you could always basically just draw right angles between the electric field and the equipotential surface. Alright, So that's always going to be true. They're always going to be perpendicular. So what that means is that in some in some questions you might be given some sort of weird equipotential surface like this. So let's say this is your equipotential and you can actually figure out where the electric field is gonna point. We know that it's always gonna be perpendicular so in other words they're gonna be at right angles like this. So th
Equipotential Surfaces - Video Tutorials & Practice Problems
Equipotential Surfaces
Video transcript
Field due to Equipotential Surfaces
Video transcript
What's up, folks? Let's work out this example problem with equipotential surfaces. So we've got these equipotential surfaces, and we're told what the potentials of each of those surfaces are, and we're also given what the spacing between them is. And using that information, we're supposed to figure out what the magnitude and the direction of the electric field due to those equipotential surfaces. Remember that there are two rules that we need to remember: the field lines are perpendicular to the equipotentials, which means that we know the electric field is going to point along this direction. But they also point in decreasing potentials. They point along decreasing potentials. So that means that the electric field has to point in this direction, which actually takes care of the second part of our problem. What is the direction of the electric field? And in more precise terms, if we know that the electric field is going to point in this, we could say is that if this angle makes a 60-degree angle with the x-axis, then that means our electric field is actually going to be pointing We're going to need to know this angle right here. So this is 30 degrees because these two things are supposed to be perpendicular. So in other words, as for the direction, the electric field will point 30 degrees below the negative x-axis. And that is the answer as for the direction.
So how do we calculate the magnitude? How do we calculate what the electric field is due to potential surfaces or changing potentials? We use the equation E = - ΔVΔx. Since we already took care of the direction, we'll focus on the magnitude. We already know that between each successive sector here or each little specific segment, we're changing 5 volts and we're doing that across the spacing of 1 centimeter, which converts to 0.01 meters. So that means that the electric field is going to have a constant magnitude of 500 volts per meter. By the way, if you're not familiar with this volts per meter unit, this actually also turns into newtons per coulomb. It's a different way to sort of express that.
So that's how you figure out what the magnitude and the direction of the electric field are due to equipotentials. First, figure out what the potential differences are, the spacings, and then just figure out your direction by going towards decreasing potentials and perpendicular to those equipotential surfaces. Alright? Let me know if you guys have any questions.
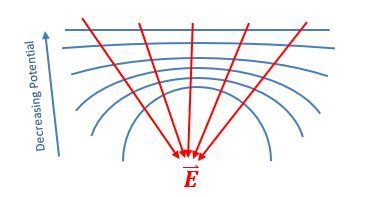
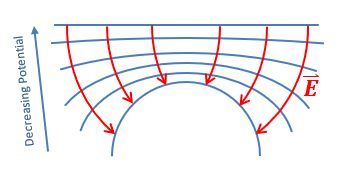
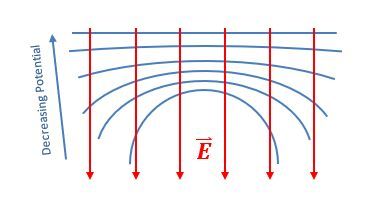
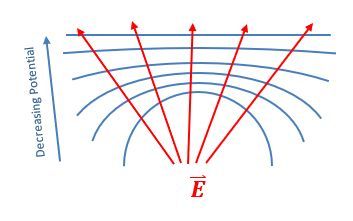
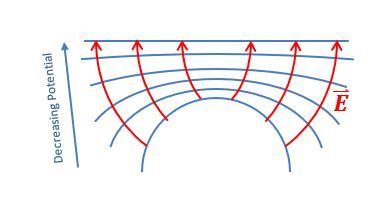
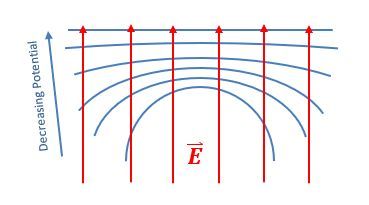
Draw the electric field that corresponds to the equipotential surfaces shown in the following figure. Note that the potential is decreasing in the upward direction.
Do you want more practice?
More setsYour Physics tutor
- A very large plastic sheet carries a uniform charge density of -6.00 nC/m^2 on one face. (a) As you move away ...
- CALC. A metal sphere with radius r_a is supported on an insulating stand at the center of a hollow, metal, sph...
- CALC. A metal sphere with radius r_a is supported on an insulating stand at the center of a hollow, metal, sph...
- CALC. A metal sphere with radius r_a is supported on an insulating stand at the center of a hollow, metal, sph...
- CALC. A metal sphere with radius r_a is supported on an insulating stand at the center of a hollow, metal, sph...
- CALC. A metal sphere with radius r_a is supported on an insulating stand at the center of a hollow, metal, sph...
- A −2.0 V equipotential surface and a +2.0 V equipotential surface are 1.0 mm apart. What is the electric field...
- (II) A metal sphere of radius r₀ = 0.35 m carries a charge Q = 0.50 μC. Equipotential surfaces are to be drawn...