Electric Flux: Videos & Practice Problems

A curved lens is placed in a uniform electric field, E. The radius of the flat circular area on the left of the lens is R.  The optical lens axis is parallel to the field's direction as shown in the image. Find out the electric flux through the curved surface.

Consider a plane surface of area 50 mm² in a uniform electric field of magnitude 25 N/C. The angle between the electric field and the normal to the surface is φ. Determine the values of angle φ for which the electric flux magnitude through the surface is i) maximum or ii) minimum.

A planar square surface with an area of 0.300 m² is immersed in a uniform electric field of magnitude 20 N/C. The normal to the square surface forms an angle of φ = 45° with the electric field. i) Calculate the electric flux (Φ_E) through the surface. ii) Why is the electric flux independent of the geometrical shape of the surface?

Given a cuboid-shaped room with uniform electric fields across all faces, a negatively charged object is placed at the center of the room as shown. The back face of the room has a missing electric field vector. Determine the direction of the missing vector, i.e., inward or outward, and calculate the minimum strength needed to maintain uniformity inside the room.

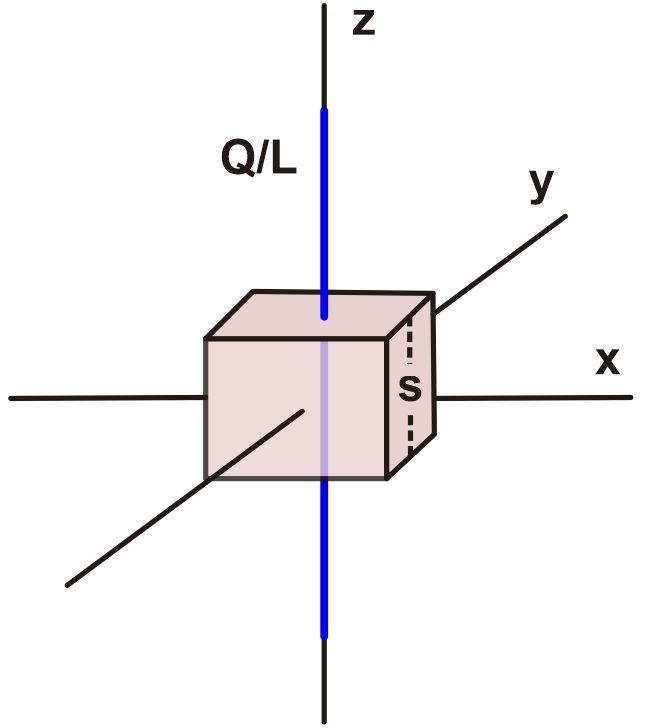
Visualize a cuboid with a side length of s, symmetrically positioned around a long line of charge. This line of charge has a linear charge density denoted by Q/L, as illustrated in the subsequent diagram. (i) Formulate an equation for the electric flux that traverses the upper surface of the cuboid, which is parallel to the xy-plane. This equation should be expressed in terms of Q/L and the dimensions of the cuboid. (ii) Subsequently, calculate the total flux passing through the cuboid. Bear in mind that the electric field penetrating the surface is not constant; it varies in both direction and intensity, depending on its relative position to the line of charge.

Consider a charge distribution that generates an electric field E = (2000r ²)r̂ N/C, where r(measured in meters) is the perpendicular distance from the z-axis to a point where r-hat points outward from the z-axis. Determine the charge enclosed within a cylindrical surface of length 2 m and radius 0.5 m, aligned along the z-axis.

An electric source is located on the bottom surface of a cube with sides measuring 2.0 cm. It generates a time-varying electric field that only passes through the top surface. The electric field is perpendicular to the bottom surface and can be described by the equation E = (2t + 1) × 10 ⁵ V/m, where t is measured in seconds. Determine the function that expresses the electric flux across the cube over time due to the electric source.

A rectangular prism is centered on an infinite line of charge with a linear charge density λ, as shown in the figure below. Determine the electric flux passing through the front face of the rectangular prism in terms of λ and the dimensions of the rectangular prism. Note that the electric field passing through the front face varies in both direction and magnitude depending on the position relative to the line of charge.

Consider a circular surface that has a radius of 5.0 cm. This surface is positioned on the plane defined by the x and y-axes. The unit vector corresponding to the area of the circular surface points towards the positive direction of the z-axis. Assuming that an electric field, represented by E = (3000 j + 1000 k) Newtons per Coulomb, is acting on this surface, calculate the electric flux that passes through this circular surface.

In the xy-plane, a rectangular surface is lying with one of its corners at the origin. The surface has dimensions of 15.0 cm parallel to the x-axis and 10.0 cm parallel to the y-axis. The unit vector normal to the surface points in the positive z-direction. An electric field of magnitude (1500/m)y N/C k is applied uniformly over the surface. What is the electric flux through it?

A tiny spherical conductor with a net charge of +5.3 nC is suspended at the center of an octahedron with a side length of 2.0 meters. What is the electric flux through one of the triangular faces of the octahedron?

Determine the electric flux passing through five different surfaces (labelled P through T) depicted in the diagram below.

A uniform magnetic field that has a magnitude strength value of about 7 x 10⁻³ T passes through a rectangle that has the following dimensions: a width that is equal to 12 cm and a height that is equal to 18 cm. What would be the magnetic flux in the face of this rectangle at an angle equivalent to 60 degrees about these field lines?

Consider having a long, thin nanowire placed along the 𝑥-axis. This nanowire has a uniform linear charge density 𝜆. Surrounding the nanowire, you have a cubic box made of a special transparent dielectric material, which allows you to observe the electric field lines without distortion. The cube has an edge length 𝐿 and is centered on the nanowire, such that the nanowire passes through the center of the cube. Consider the face parallel to the xz-plane. Define the small area 𝑑𝐴 as a strip of width 𝑑𝑧 and length 𝐿 with the vector pointing in the -y-direction. Utilize the established electric field of a wire to determine the electric flux 𝑑Φ through this small area. Express your formula in terms of the variable 𝑧 and relevant constants, ensuring it does not explicitly include any angles.