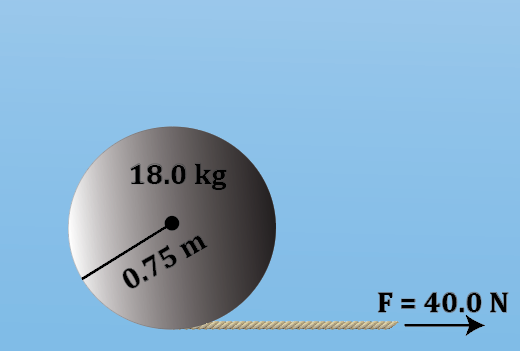
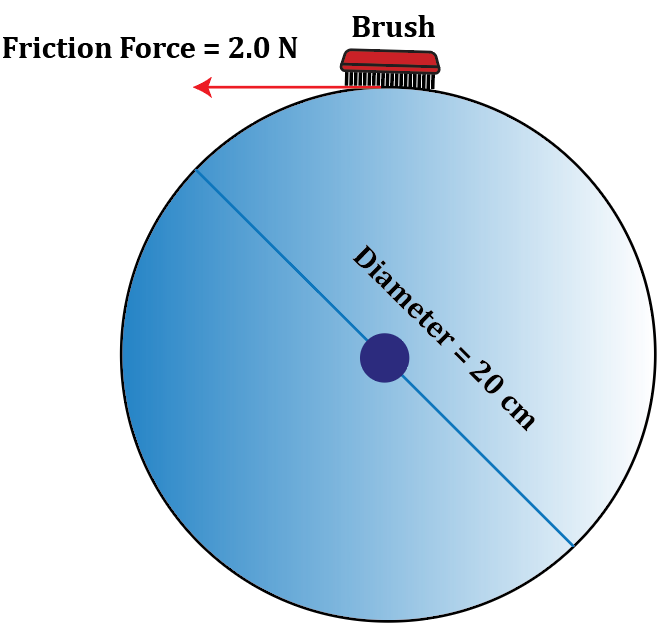
14. Torque & Rotational Dynamics
Torque & Acceleration (Rotational Dynamics) Practice Problems
14. Torque & Rotational Dynamics
Torque & Acceleration (Rotational Dynamics) Practice Problems
33 problems