1. Intro to General Chemistry
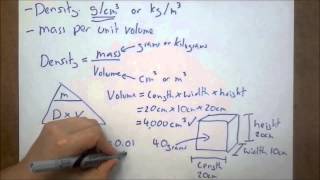
Density of Geometric Objects
Problem 73
Textbook Question
Textbook QuestionWhat is the density of lithium metal in g/cm3 if a cylindrical wire with a diameter of 2.40 mm and a length of 15.0 cm has a mass of 0.3624 g?
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
3mPlay a video:
877
views
Was this helpful?
Related Videos
Related Practice
Showing 1 of 10 videos