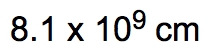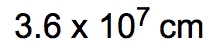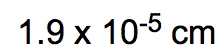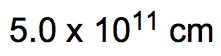1. Intro to General Chemistry
Dimensional Analysis
1. Intro to General Chemistry
Dimensional Analysis - Video Tutorials & Practice Problems

Get help from an AI Tutor
Ask a question to get started.
We use dimensional analysis as a fail proof process to convert from one unit to another.
Dimensional Analysis
1
concept
Dimensional Analysis
Video duration:
6mPlay a video:
Using Dimensional Analysis, start with the given amount and obtain the end amount by using conversion factors.

2
example
Dimensional Analysis Example 1
Video duration:
4mPlay a video:
3
Problem
ProblemIf the distance between Washington, D.C. and New York City is 224.9 miles, determine the distance in centimeters.
A
B
C
D
E
4
Problem
ProblemA large scale ranching farm possesses 22.5 million acres with 5.0 x 10-5 % reserved for housing. Determine how many square kilometers this area for dwelling represents. (1 km = 0.6214 miles) (1 mile = 5280 ft) (1 acre = 43,560 ft2)
A
9.2 km2
B
0.115 km2
C
1.8 km2
D
0.046 km2
Do you want more practice?
More setsYour General Chemistry tutor
Additional resources for Dimensional Analysis
PRACTICE PROBLEMS AND ACTIVITIES (121)
- Consider the jar of jelly beans in the photo. To get an estimate of the number of beans in the jar you weigh s...
- A person runs at a pace of 6.52 mi/hr. How long does it take the person to run a 15.0 km race? (1 mi = 1.61 km...
- The radius of an atom of tungsten (W) is about 2.10 A . (c) If the atom is assumed to be a sphere, what is the...
- The radius of an atom of tungsten (W) is about 2.10 A . (b) How many tungsten atoms would have to be lined up ...
- The radius of an atom of tungsten (W) is about 2.10 A . (a) Express this distance in nanometers (nm).
- The radius of an atom of copper (Cu) is about 140 pm. (c) If you assume that the Cu atom is a sphere, what is...
- If Avogadro's number of pennies is divided equally among the 321 million men, women, and children in the Unite...
- The Ogallala aquifer described in the Closer Look box in Section 18.3, provides 82% of the drinking water for ...
- The physical fitness of athletes is measured by 'VO2 max,' which is the maximum volume of oxygen consumed by a...
- The estimated concentration of gold in the oceans is 1.0 * 10^-11 g/mL. (b) Assuming that the volume of the oc...
- (d) Bamboo can grow up to 60.0 cm/day. Convert this growth rate into inches per hour.
- (b) The lung capacity of the blue whale is 5.0 * 103 L. Convert this volume into gallons.
- (a) A bumblebee flies with a ground speed of 15.2 m/s. Calculate its speed in km/hr.
- (a) The speed of light in a vacuum is 2.998 * 108 m>s. Calculate its speed in miles per hour.
- (d) An individual suffering from a high cholesterol level in her blood has 242 mg of cholesterol per 100 mL o...
- (c) The Vehicle Assembly Building at the Kennedy Space Center in Florida has a volume of 3,666,500 m3. Convert...
- (b) The Sears Tower in Chicago is 1454 ft tall. Calculate its height in meters.
- Perform the following conversions: (f) 0.02500 ft3 to cm3.
- Perform the following conversions: (e) 22.50 gal/min to L/s
- Perform the following conversions: (d) 0.510 in./ms to km/hr
- Perform the following conversions: (c) $1.89/gal to dollars per liter
- Perform the following conversions: (b) 0.0550 mi to m
- Perform the following conversions: (a) 5.00 days to s
- Carry out the following conversions: (e) $3.99/lb to dollars per kg
- Carry out the following conversions: (d) 1.955 m3 to yd3
- Carry out the following conversions: (a) 0.105 in. to mm
- (a) How many liters of wine can be held in a wine barrel whose capacity is 31 gal?
- (c) If an automobile is able to travel 400 km on 47.3 L of gasoline, what is the gas mileage in miles per gall...
- (d) When the coffee is brewed according to directions, a pound of coffee beans yields 50 cups of coffee 14 cup...
- (b) The recommended adult dose of Elixophyllin®, a drug used to treat asthma, is 6 mg/kg of body mass. Calcula...
- Complete the table. a. 355 km>s _____cm>s _____m>ms b. 1228 g>l _____g>ml _____kg>ml c. 554 ...
- (d) In March 1989, the Exxon Valdez ran aground and spilled 240,000 barrels of crude petroleum off the coast o...
- (a) If an electric car is capable of going 225 km on a single charge, how many charges will it need to travel ...
- Complete the table. a. 355 km>s _____cm>s _____m>ms b. 1228 g>l _____g>ml _____kg>ml c. 554 ...
- Gold can be hammered into extremely thin sheets called gold leaf. An architect wants to cover a 30 m * 25 m ce...
- What is the volume in L of a cube with an edge length of 7.0 dm?
- What is the volume in mL of a cube with an edge length of 2.5 cm?
- The distance from Earth to the Moon is approximately 240,000 mi. (d) Earth travels around the Sun at an aver...
- The distance from Earth to the Moon is approximately 240,000 mi. (c) The speed of light is 3.00 * 108 m>s...
- The distance from Earth to the Moon is approximately 240,000 mi. (a) What is this distance in meters?
- The distance from Earth to the Moon is approximately 240,000 mi. (b) The peregrine falcon has been measured as...
- The U.S. quarter has a mass of 5.67 g and is approximately 1.55 mm thick. (c) How much money would this stack ...
- The U.S. quarter has a mass of 5.67 g and is approximately 1.55 mm thick. (a) How many quarters would have to ...
- In the United States, water used for irrigation is measured in acre-feet. An acre-foot of water covers an acr...
- The American Dental Association recommends that an adult female should consume 3.0 mg of fluoride (F - ) per d...
- In 1986 an electrical power plant in Taylorsville, Georgia, burned 8,376,726 tons of coal, a national record a...
- In January 2006, the New Horizons space probe was launched from Earth with the mission to perform a flyby stud...
- A 40-lb container of peat moss measures 14 * 20 * 30 in. A 40-lb container of topsoil has a volume of 1.9 gal....
- The total rate at which power is used by humans worldwide is approximately 15 TW (terawatts). The solar flux a...
- The diameter of a rubidium atom is 495 pm We will consider two different ways of placing the atoms on a surfac...
- (a) Assuming the dimensions of the nucleus and atom shown in Figure 2.10, what fraction of the volume of the a...
- Very small semiconductor crystals, composed of approximately 1000 to 10,000 atoms, are called quantum dots. ...
- A period at the end of sentence written with a graphite pen-cil has a diameter of 1 mm. If the period represen...
- Perform each unit conversion. a. 1.4 in to mm b. 116 ft to cm c. 1845 kg to lb d. 815 yd to km
- A 1/4-inch-thick lead sheet is used for protection from medi-cal X rays. If a single lead atom has a diameter ...
- Carry out the following conversions. (a) How many grams of meat are in a quarter-pound hamburger (0.25 lb)?
- Carry out the following conversions. (b) How tall in meters is the Willis Tower, formerly called the Sears To...
- Carry out the following conversions. (c) How large in square meters is the land area of Australia (2,941,526 ...
- In 2009, a team from Northwestern University and Western Washington University reported the preparation of a n...
- Perform each unit conversion. a. 1.4 in to mm b. 116 ft to cm c. 1845 kg to lb d. 815 yd to km
- Perform each unit conversion. a. 1.4 in to mm b. 116 ft to cm c. 1845 kg to lb d. 815 yd to km
- Perform each unit conversion. a. 1.4 in to mm b. 116 ft to cm c. 1845 kg to lb d. 815 yd to km
- A period at the end of sentence written with a graphite pen-cil has a diameter of 1 mm. How many carbon atoms ...
- Convert the following quantities into SI units with the correct number of significant figures. (c) 0.5521 gal
- Convert the following quantities into SI units with the correct number of significant figures. (e) 978.3 yd3
- Convert the following quantities into SI units with the correct number of significant figures. (b) 66.31 lb
- Convert the following quantities into SI units with the correct number of significant figures. (a) 5.4 in.
- Convert the following quantities into SI units with the correct number of significant figures. (d) 65 mi/h
- Convert the following quantities into SI units with the correct number of significant figures. (f) 2.380 mi^2
- A runner wants to run 10.0 km. Her running pace is 7.5 mi per hour. How many minutes must she run?
- A cyclist rides at an average speed of 18 mi per hour. If she wants to bike 212 km, how long (in hours) must s...
- The world record for the women's outdoor 20,000-meter run, set in 2000 by Tegla Loroupe, is 1:05:26.6 (seconds...
- In a sunny location, sunlight has a power density of about 1 kW/m2. Photovoltaic solar cells can convert this ...
- In the United States, the emissions limit for carbon monoxide in motorcycle engine exhaust is 12.0 g of carbon...
- A certain European automobile has a gas mileage of 17 km>L. What is the gas mileage in miles per gallon?
- The volume of water used for crop irrigation is measured in acre-feet, where 1 acre-foot is the amount of wate...
- A gas can holds 5.0 gal of gasoline. Express this quantity in cm3.
- The height of a horse is usually measured in hands instead of in feet, where 1 hand equals 1/3 ft (exactly). ...
- The height of a horse is usually measured in hands instead of in feet, where 1 hand equals 1/3 ft (exactly). ...
- Weights in England are commonly measured in stones, where 1 stone = 14 lb. What is the weight in pounds of a p...
- Concentrations of substances dissolved in solution are often expressed as mass per unit volume. For example, n...
- The average U.S. farm occupies 435 acres. How many square miles is this? (1 acre = 43,560 ft2, 1 mile = 5280 f...
- Administration of digitalis, a drug used to control atrial fibrilla-tion in heart patients, must be carefully ...
- Total U.S. farmland occupies 954 million acres. How many square miles is this? (1 acre = 43,560 ft2, 1 mi = 52...
- An acetaminophen suspension for infants contains 80 mg> 0.80 mL suspension. The recommended dose is 15 mg&g...
- Among many alternative units that might be considered as a measure of time is the shake rather than the second...
- Which is larger in each pair, and by approximately how much? (d) A centimeter or an inch
- Which is larger in each pair, and by approximately how much? (b) A mile or a kilometer
- An ibuprofen suspension for infants contains 100 mg>5.0 mL suspension. The recommended dose is 10 mg>kg ...
- The density of polystyrene, a plastic commonly used to make CD cases and transparent cups, is 0.037 lbs/in3. C...
- There are exactly 60 seconds in a minute, exactly 60 minutes in an hour, exactly 24 hours in a mean solar day,...
- Determine the number of picoseconds in 2.0 hours.
- The density of polypropylene, a plastic commonly used to make bottle caps, yogurt containers, and carpeting, i...
- A large tanker truck for carrying gasoline has a capacity of 3.4 * 104 L. (a) What is the tanker's capacity i...
- A large tanker truck for carrying gasoline has a capacity of 3.4 * 104 L. (b) If the retail price of gasoline...
- A 1.0-ounce piece of chocolate contains 15 mg of caffeine, and a 6.0-ounce cup of regular coffee contains 105 ...
- A penny has a thickness of approximately 1.0 mm. If you stacked Avogadro's number of pennies one on top of the...
- Consider the stack of pennies in the previous problem. How much money (in dollars) would this represent? If th...
- Answer the following questions. (a) An old rule of thumb in cooking says: 'A pint's a pound the world around.'...
- Answer the following questions. (b) There are exactly 640 acres in 1 square mile. How many square meters are i...
- A bag of Hershey's Kisses contains the following information: Serving size: 9 pieces = 41 g Calories per servi...
- Hydrogen cyanide, HCN, is a poisonous gas. The lethal dose is approximately 300 mg HCN per kilogram of air wh...
- The source of oxygen that drives the internal combustion engine in an automobile is air. Air is a mixture of g...
- The value of the euro was recently $1.15 U.S., and the price of 1 liter of gasoline in France is 1.42 euro. Wh...
- Ocean currents are measured in Sverdrups (sv) where 1 sv = 109 m3/s. The Gulf Stream off the tip of Florida, f...
- Ocean currents are measured in Sverdrups (sv) where 1 sv = 109 m3/s. The Gulf Stream off the tip of Florida, f...
- Ocean currents are measured in Sverdrups (sv) where 1 sv = 109 m3/s. The Gulf Stream off the tip of Florida, f...
- The density of iron is 7.86 g>cm3. What is its density in pounds per cubic inch (lb>in3)?
- The element gallium (Ga) has the second-largest liquid range of any element, melting at 29.78 °C and boiling a...
- The Toyota Prius, a hybrid electric vehicle, has an EPA gas mileage rating of 52 mi>gal in the city. How ma...
- The Honda Insight, a hybrid electric vehicle, has an EPA gas mileage rating of 41 mi>gal in the city. How m...
- A sample of gaseous neon atoms at atmospheric pressure and 0 °C contains 2.69 * 1022 atoms per liter. The atom...
- The diameter of a hydrogen atom is 212 pm. Find the length in kilometers of a row of 6.02 * 1023 hydrogen atom...
- The world's record in the 100-m dash is 9.69 s, and in the 100-yd dash it is 9.21 s. Find the speed in mi>h...
- The daily recommended intake of calcium for an average adult is 1,000 mg. There is 125 mg of calcium in 100 gr...
- A length of #8 copper wire (radius = 1.63 mm) has a mass of 24.0 kg and a resistance of 2.061 ohm per km (Ω &g...
- An infant acetaminophen suspension contains 80 mg/0.80 mL suspension. The recommended dose is 15 mg/kg body we...
- Assuming the diameters of the nucleus and atom are 10−4 å and 1 - 5 å correspondingly, what fraction of the vo...
- If an object has a density of 8.65 g/cm3, what is its density in units of kg/m3?
- If a cyclist rides at an average of 18 miles per hour
- An unknown substance has a density of 10.2 g/cm3, what is its density in kg/m3?