9. Quantum Mechanics
Quantum Numbers: Spin Quantum Number
9. Quantum Mechanics
Quantum Numbers: Spin Quantum Number - Video Tutorials & Practice Problems

Get help from an AI Tutor
Ask a question to get started.
The Spin Quantum Number deals with the spin of an electron within an orbital.
Spin Quantum Number
1
concept
Quantum Numbers: Spin Quantum Number
Video duration:
1mPlay a video:
2
example
Quantum Numbers: Spin Quantum Number Example 1
Video duration:
1mPlay a video:
3
Problem
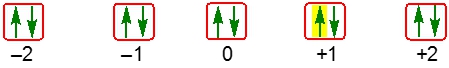
ProblemSelect the correct quantum numbers for the highlighted electrons in a set of 5d orbitals.
A
n = 5, l = 3, ml = – 4, ms = +1/2
B
n = 4, l = 4, ml = +1, ms = +1/2
C
n = 5, l = 2, ml = +1, ms = +1/2
D
n = 5, l = 5, ml = – 2, ms = +1/2
E
n = 5, l = 2, ml = +5, ms = +1/2
4
Problem
ProblemWhich of the following set of quantum numbers is possible?
A
n = 8, l = 3 , mll = 0, ms = 0
B
n = 7, l = 2, ml = 1, ms = –1/2
C
n = 9, l = 1, ml = – 2, ms = +1/2
D
n = 3, l = 0, ml = +3, ms = +1/2
E
n = 4, l = 2, ml = –2, ms = +1
5
Problem
ProblemWhich of the following set of quantum numbers is possible for an electron in a set of 6f orbitals?
A
n = 6, l = 3 , mll = 0, ms = 0
B
n = 6, l = 2, ml = 1, ms = –1/2
C
n = 9, l = 1, ml = – 2, ms = +1/2
D
n = 6, l = 3, ml = 0, ms = +1/2
E
n = 4, l = 2, ml = –2, ms = +1
6
Problem
ProblemWhich of the following statements is false?
A
If an electron has n = 2, it possesses only s and p orbitals.
B
Each orbital within a given atom can hold up to 2 electrons.
C
The second shell of an atom possesses d orbitals.
D
A set of f orbitals can hold a maximum of 14 electrons.
E
The first energy level contains only s orbitals.
Do you want more practice?
More setsYour General Chemistry tutor
Additional resources for Quantum Numbers: Spin Quantum Number
PRACTICE PROBLEMS AND ACTIVITIES (13)
- A certain orbital of the hydrogen atom has n = 4 and l = 3. (b) What are the possible values of ms for the orb...
- Give the allowable combinations of quantum numbers for each of the following electrons. (a) A 4s electron (b) ...
- Assign a set of four quantum numbers to each electron in oxygen.
- Determine whether each of the following sets of quantum numbers for the hydrogen atom are valid. If a set is ...
- Which set of three quantum numbers does not specify an orbital in the hydrogen atom?
- Quantum numbers arise naturally from the mathematics used to describe the possible states of an electron in an...
- Which of the following sets of quantum numbers is not allowed?
- Which of the following set of quantum numbers (ordered n, ℓ, mℓ, ms) are possible for an electron in an atom?
- Identify which sets of quantum numbers are valid for an electron. each set is ordered (n,ℓ,mℓ,ms).
- Which one of the following sets of quantum numbers is not possible?
- Identify the set of quantum numbers of each of the following elements
- Select the acceptable sets of quantum numbers in an atom.
- What rule/principle states that electrons fill orbitals from lowest energy to highest energy?